Question

A padlock has a four-digit code that includes digits from 0 to 9, inclusive. What is the probability that the code does not consist of all odd digits if the same digit is not used more than once in the code?

120 out of 5,040
120 out of 3,024
2,904 out of 3,024
4,920 out of 5,040

Answer 1

It's D

Explanation:

Edge 2022

Answer 2

Use the theoretical definition of probability:

`Pr=\frac{\text{number of all favorable outcome}}{\text{number of all possible outcomes}}.`

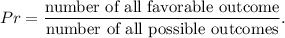
1. Find the probability that the code consists of all odd digits if the same digit is not used more than once in the code.

The number of all possible outcomes is

`10\cdot 9\cdot 8\cdot 7=5040.`

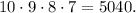
The number of codes in which all digits are odd is

`5\cdot 4\cdot 3\cdot 2=120.`

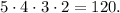
Then,

`Pr(\text{all digits in code are odd})=(120)/(5040).`

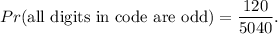
2. Find the probability that the code does not consist of all odd digits if the same digit is not used more than once in the code:

`Pr(\text{not all digits are odd})=1-Pr(\text{all digits in code are odd})=1-(120)/(5040)=(4920)/(5040).`

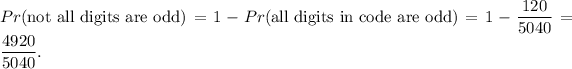
Answer: correct choice is D