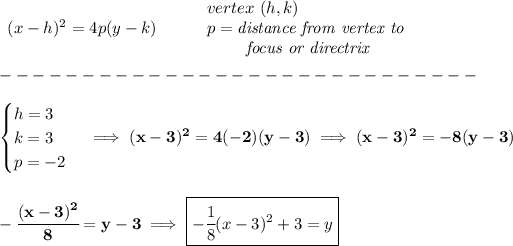
so hmmm check the picture below
so... the vertex is "p" distance from the focus and the directrix, thus, the vertex is really half-way between both
in this case, 2 units up from the focus or 2 units down from the directrix, and thus it lands at 3,3
now, the "p" distance is 2, however, the directrix is up, the focus point is below it, the parabola opens towards the focus point, thus, the parabola is opening downwards, and the squared variable is the "x"
because the parabola opens downwards, "p" is negative, and thus, -2
now, let's plug all those fellows in then