Answer:
Volume of cone A = 4 pi cubic inches , Volume of cone B= 16 pi cubic inches.
Volume of cone B is 4 times of volume of cone A.
Explanation:
Given : Cone A has a radius of 2 inches and a height of 3 inches. In cone B, the height is the same, but the radius is doubled.
To find : Calculate the volume of both cones. Which statement is accurate.
Solution : We have given two cones A and cone B.
Radius of cone A = 2 inches.
Height of cone A= 3 inches.
Radius of cone B= 4 inches. ( double of radius of cone A)
Height of cone A= 3 inches.
Volume of cone =
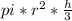 .
.
Volume of cone A =
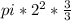 .
.
Volume of cone A = 4 pi cubic inches.
Volume of cone B =
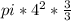 .
.
Volume of cone B= 16 pi cubic inches.
Volume of cone B is 4 times of volume of cone A.
Therefore, Volume of cone A = 4 pi cubic inches , Volume of cone B= 16 pi cubic inches.
Volume of cone B is 4 times of volume of cone A.