Question

water from melting snow drips from a roof at a height of 40ft. the function h=-16t^2+40 gives the approximate height h in feet of a drop of water t seconds after it falls. graph the function

Answer 1

The given function is

`f(x)=-16t^2+40`

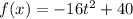
First, we find the vertex V(h,k), where

`h=-(b)/(2a)`

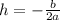
We know that a = -16 and b = 0, so

`h=-(0)/(2\cdot(-16))=0`

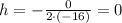
Then, we find k evaluating the function when x = 0.

`k=f(x)=-16(0)^2+40=40`

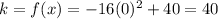
The vertex is V(0, 40).

Then, we find the intercepts of the function when y = 0.

`\begin{gathered} -16t^2+40=0 \\ -16t^2=-40 \\ t^2=-(40)/(-16) \\ t=\sqrt[]{2.5} \\ t\approx\pm1.58 \end{gathered}`

The intercepts are (-1.58, 0) and (1.58, 0).

Now, we use all three points to graph the function.

The image below shows the graph of the function:

In the image, we used t = x.