Question

A population of 80,000 toads is expected to shrink at a rate of 9.2% per year. What will be the toad population be in 20 years?

Answer 1

Using the formula:

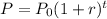`P = P_0(1+r)^t` .....[1]

where,

P is the population after t years

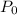`P_0` is the initial population

r is the rate (in decimal)

As per the statement:

A population of 80,000 toads is expected to shrink at a rate of 9.2% per year.

⇒Initial population(
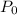`P_0`) = 80,000 toad

and

r = -9.2% = -0.092

We have to find the toad population be in 20 years.

t = 20 years

Substitute the given values in [1], we have;

`P = 8000 \cdot (1-0.092)^(20)`

⇒
`P = 8000 \cdot (0.908)^(20) = 80000 \cdot 0.145116559`

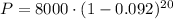
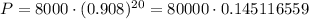
Simplify:

`P = 11609.3247 \approx 11609`

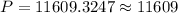
Therefore, about 11609 will be the toad population be in 20 years.

Answer 2

P=80,000×(1−0.092)^(20)
p=11,609.3