Answer:
Local maximum: (-1/5, -10)
Local minimum: (1/5, 10)
Step-by-step explanation:
Given the below function;
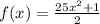
To determine the relative maxima of the above function using the First Derivative Test, we'll follow the below steps;
Step 1: Determine the derivative of f(x) using the Quotient rule;
Let u = 25x^2 + 1
u' = 50x
Let v = x
v' = 1
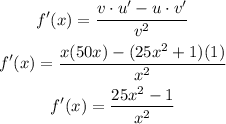
Step 2: Determine the critical points by equating f(x) to zero and solving for x;
![\begin{gathered} (25x^2-1)/(x^2)=0 \\ 25x^2-1=0 \\ 25x^2=1 \\ x^2=(1)/(25) \\ x=\pm\sqrt[]{(1)/(25)} \\ x=\pm(1)/(5) \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/hpnk3lstbw22lqbtsshq.png)
Step 3: Since the critical points are at -1/5 and 1/5, we'll check if the first derivative changes signs around these points by picking numbers from different intervals as seen below;
From negative infinity to -1/5, let's pick -1;
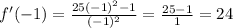
From -1/5 to 1/5, let's pick 0.1;
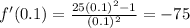
From 1/5 to infinity; let's pick 1;
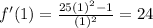
We can see from the above that the first derivative changes from positive to negative around x = -1/5, it signifies that this critical point is a local maximum.
It also changes from negative to positive around x = 1/5, it signifies that this critical point is a local minimum.
Let's go ahead and determine the actual points at which the function has the local minimum and maximum by evaluating f(x) at the critical points;
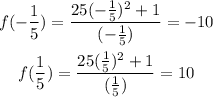
Therefore, the function f(x) has a local maximum at (-1/5, -10) and a local minimum at (1/5, 10)