Question

2x - y - 4 = 0

3x + y - 9 = 0

What is the solution set of the given system?

a. {(6/5, 13/5)}
b. {(13/5, 6/5)}
c. {(5, 6)}
d. {(6, 5)}

Answer 1

hello :
2x - y - 4 = 0 ....(1)
3x + y - 9 = 0 ....(2)
(1)+(2) : 5x-13 =0
5x=13
x=13/5
subsct in (2) :3(13/5)+y-9=0
y = 9-39/5
y=6/5
answer : b. {(13/5, 6/5)}

Answer 2

Option B.

Explanation:

The given equations of the system are

2x - y - 4 = 0

2x - 4 = y ------(1)

3x + y - 9 = 0

3x + y = 9

y = 9 - 3x ------(2)

By replacing the value of y from equation (1) to equation (2)

2x - 4 = 9 - 3x

2x = 4 + 9 - 3x

2x = 13 - 3x

2x + 3x = 13

5x = 13

x =
`(13)/(5)`

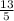
Now we plug in the value of x in the equation (1)

`y=9-(3* (13)/(5))`

`y=9-((39)/(5))`

`y=(45-39)/(5)`

`y=(6)/(5)`

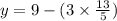
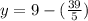
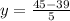
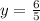
Therefore, solution of the given system is
`[(13)/(5),(6)/(5)]`.

Option B. is the answer.