Question

For the transformation T, what is T-1? T : (x, y) (x + 4, y + 3) T-1: (x, y)

Answer 1

The inverse transformation of T: (x,y) =(x+4 , y+3) is
`T^(-1)=(x-4,y-3)`.

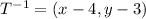Explanation:

The given transformation T takes some point (x,y) and turns it into (x+4, y+3). Here, x coordinate is increased by 4 and y coordinate is increased by 3 .

To find the inverse of transformation, simply reverse the operations.

Now T inverse takes the point (x,y) and turns it into as follows,

previously x is increased by 4 we now decrease x to 4 and y previously increased by 3 we now decrease y to 3 .

now we get , the answer as
`T^(-1)=(x-4,y-3)`

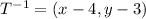
Thus, the inverse transformation of T: (x,y) =(x+4 , y+3) is
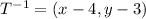`T^(-1)=(x-4,y-3)`.

Answer 2

The answer is:
T : (x, y) (x + 4, y + 3)
T⁻¹: (x, y) (x - 4, y - 3)

The basic principle is to find the inverse function of F, such that T*T⁻¹ = T⁻¹ * T = I.