Question

How long a time t will it take for the 133 54xe to decay so that eventually its activity decreases by a factor of 1024?

Answer 1

This is an incomplete question, here is a complete question.

The radioactive isotope
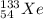`^(133)_(54)Xe` is used in pulmonary respiratory studies to image the blood flow and the air reaching the lungs. The half-life of this isotope is 5 days .

How long a time t will it take for the
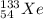`^(133)_(54)Xe` to decay so that eventually its activity decreases by a factor of 1024?

Express your answer numerically in days.

Answer : The time passed in days is, 50 days

Explanation :

Half-life = 5 days

First we have to calculate the rate constant, we use the formula :

`k=(0.693)/(t_(1/2))`

`k=\frac{0.693}{5\text{ days}}`

`k=0.1386\text{ days}^(-1)`

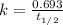
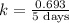
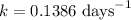
Now we have to calculate the time passed.

Expression for rate law for first order kinetics is given by:

`t=(2.303)/(k)\log(a)/(a-x)`

where,

k = rate constant =
`0.1386\text{ years}^(-1)`

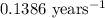
t = time passed by the sample = ?

a = initial amount of the reactant = 1

a - x = amount left after decay process =
`(1)/(1024)`

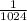
Now put all the given values in above equation, we get

`t=(2.303)/(0.1386)\log(1)/(((1)/(1024)))`

`t=50.02\text{ days}\approx 50\text{ days}`

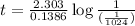
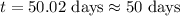
Therefore, the time passed in days is, 50 days

Answer 2

Base on my research the radioactive isotope X-133 has a half-life of 5 days. So base on the given it decreases by a factor of 1024, it represents 10 halvings. To get how long will it take for the 133 54xe to decay, just multiply 5 days with 10 halvings. The answer is 50 days for the 133 54xe to decay.