We can express the area (A) of a trapezoid as the product of the average between the bases (a and b) and the height (h).
Then, we can write:
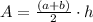
We then, can express the height as:
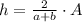
Replacing with the known values (a=119 cm, b=65 cm, A=6578 cm^2), we get:
![\begin{gathered} h=\frac{2}{119\operatorname{cm}+65\operatorname{cm}}\cdot6578\operatorname{cm}^2 \\ h=\frac{2}{184\operatorname{cm}}\cdot6578\operatorname{cm} \\ h=\frac{6578\operatorname{cm}^2}{92\operatorname{cm}} \\ h=71.5\operatorname{cm} \end{gathered}]()
Answer: the height of the trapezoid is 71.5 cm.