Question

In the circle provided, what is the value of x?

Answer 1

x = 4

Step-by-step explanation:

Intersecting tangent- secant theorem says that if we have

then

`AB^2=BC*BD`

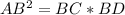
Now in our case,

AB = 8, BC = x, and CD = 12 + x; therefore, the above formula gives

`8^2=x(x+12)`

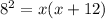
Expanding the above gives

`64=x^2+12x`

subtracting 64 from both sides gives

`x^2+12x-64=0`

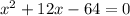
Using the quadratic formula, the two solutions we get are:

`x=(-12\pm√(12^2-4(1)(-64)))/(2)`

which we evaluate to get:

`x=(-12\pm20)/(2)`

which gives us two solutions:

`\begin{gathered} x=-16 \\ x=4 \end{gathered}`

Since a length cannot be a negative number, x = 4 is our relevant solution.