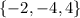Given;
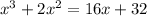
To find: The equation in factored form and solution set.
Step-by-step explanation:
Given equation can be written as
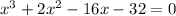
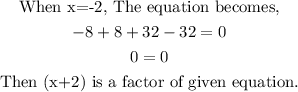
By using the factor (x+2), We proceed with the synthetic division
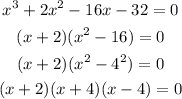
Therefore, the equation is factored form:
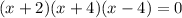
Then the solution set is