Question

Explain the error a student is asked to find when the value of an investment of $5200 is an account that earns 4.2% annual interest compounded quarterly reaches $16,500. The student uses the model V(t)=5200(1.014)^3t and finds that the investment reaches a value of $16,500 after approximately 27.7 years. Fine and correct the students error.

Answer 1

We are given the following information

Investment = $5200

Annual interest rate = 4.2% = 0.042

Final amount = $16,500

Number of years = 27.7 years

Number of compoudings = quartely = 4

The student uses the following model

`V(t)=5200(1.014)^(3t)`

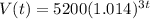
The general formula for compound interest is given by

`V(t)=P(1+(r)/(n))^(nt)`

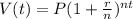
As you can see, the number of compoundings is incorrect (3 vs 4)

The interest rate is also incorrect.

Let us substitute the given values into the above formula

`\begin{gathered} V(t)=P(1+(r)/(n))^(nt) \\ V(t)=5200(1+(0.042)/(4))^(4\cdot27.7) \\ V(t)=5200(1+0.0105)^(110.8) \\ V(t)=5200(1.0105)^(110.8) \\ V(t)=\$16543.5^{} \end{gathered}`

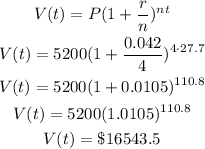
Therefore, the final amount is approximately $16,543.5