Question

A teacher takes her class and some of the children's parents on a field trip to a museum She purchased a total of 56 tickets for a total of $284. If children's tickets each cost $3 and adult tickets each cost $7 how many children and how many adults went on the field trip?

Answer 1

Let x and y be the number of children and adults in the group, respectively. Therefore, the two equations are

`\begin{gathered} x+y=56\to\text{ 56 tickets in total} \\ 3x+7y=284\to\text{ total cost of the tickets} \end{gathered}`

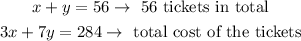
Solve the system of equations as shown below

`\begin{gathered} x=56-y \\ \Rightarrow3(56-y)+7y=284 \end{gathered}`

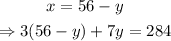
Solving the former equation for y,

`\begin{gathered} \Rightarrow168-3y+7y=284 \\ \Rightarrow4y=116 \\ \Rightarrow y=29 \end{gathered}`

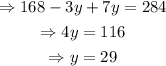
Finding x,

`\begin{gathered} \Rightarrow x=56-29=27 \\ \Rightarrow x=27 \end{gathered}`

The answer is 27 children and 29 adults in total.