Answer:
Volume(V) of a right square pyramid is given by:
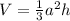
where,
a is the base length and h is the height respectively.
As per the statement:
A square pyramid that has a volume of 12 cubic feet and a base length of 3 feet.
⇒V = 12 cubic feet and a = 3 feet
Substitute these in [1] we have';
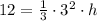
⇒
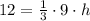
⇒
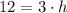
Divide both sides by 3 we have;
4 = h
or
h = 4 feet
Therefore, the height of of a square pyramid is, 4 feet