Question

Draw the image of quadrilateral EJMX aftertranslating it across the given vector andreflecting it across the y-axis. Make sure tolabel the final image appropriately

Answer 1

We can see that the quadrilateral is located in the points:

M(1, -2)

X(3, -3)

E(-2, -4)

J(-2, -3)

After this we can see that the vector represents the following rule:

`(x,y)\to(x+2,y+3)`

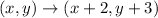
So, we calculate the new coordinates M'X'E'J':

M'(3, 1)

x'(5, 0)

E'(0, -1)

J'(0, 0)

So, the first translation is:

After this, we reflect across the y-axis to obtain M''X''E''J'', that is following the rule:

`(x,y)\to(-x,y)`

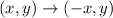
So, we find the new points:

M''(-3, 1)

X''(-5, 0)

E''(0, -1) = E'(0, -1)

J''(0, 0) = J'(0, 0)

Finally, the y-axis reflection is:

And that is the first and final positions of the points.

***Here all of the transformations in one image: