Answer: 27783
Explanation:
Given : The number of toppings for pizza= 21
If repetition of toppings is allowed, then the number of different 3-topping combinations will be :-
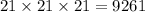
Also, the number of choices for crust = 3
Then, the number if different 3-topping pizzas can be made if toppings can be selected more than once will be :_
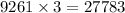
Hence, the number of different 3-topping pizzas can be made = 27783