Answer: It will take 10 hours.
Explanation:
Given: Distance traveled by Ruby in
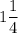 hours =
hours =
 of Total trip.
of Total trip.
i.e. Time taken to complete
 of Total trip=
of Total trip=
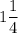 hours
hours
Time taken to cover the total trip = Reciprocal of
 x (
x (
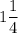 )
)
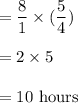
Therefore , it will take 10 hours to complete the trip.