Answer: 0.1587
Explanation:
Given: Mean :
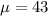
Standard deviation :
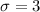
Sample size : n = 90
We know that , the value of z is given by :-
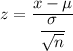
For x=4.2
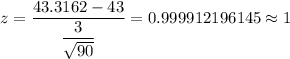
The p value =
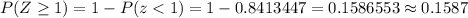
Hence, the probability that a sample of 90 students will have a mean score of at least 43.3162= 0.1587