Question

Point C is the center of the circle. The measure of angle ACB is 3x + 120. Arc AB measures 65x – 4. Find the measure of arc AB.

Answer 1

In a circle The measurement of a CENTRAL ANGLE (vertex C) is equal to the measurement of the arc intercepted:

65x-4 = 3x+120 ===> 62x=124 & x= 2, hence the arc = 2

Answer 2

The measure of arc AB is 126°.

Explanation:

It is given that point C is the center of the circle. The measure of angle ACB is 3x + 120. Arc AB measures 65x – 4.

The measure of central angle is equal to the measure of arc.

`3x+120=65x-4`

Solve this equation for x.

`120+4=65x-3x`

`124=62x`

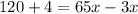
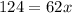
Divide both the sides by 62.

`(124)/(62)=x`

`2=x`

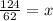
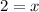
The value of x is 2.

The measure of arc AB is

`65x-4=65(2)-4\Rightarrow 130-4=126`

Therefore the he measure of arc AB is 126°.