Question

A wedding tent is built in the shape of a right rectangular prism topped with a rectangular pyramid. The dimensions of the prism are 32 ft by 35 ft by 9 ft, and the height of the pyramid is 4 ft. Find the total volume of the tent. Round your answer to the nearest tenth if necessary. (Note: diagram is not drawn to scale.)

Answer 1

11573.3 cubic feet

Explanations:

The given tent is made up of a rectangular pyramid and prism. The formula for calculating the volume of the tent is expressed as:

`V=volume\text{ of pyramid + volume of prism}`

Find the volume of the prism

`\begin{gathered} Vol.\text{ of prism}=Base\text{ area }* Height \\ Vol.\text{ of prism}=(35*32)*9 \\ Vol.\text{ of prism}=1120*9 \\ Vol.\text{ of prism}=10,080ft^3 \end{gathered}`

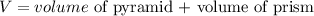
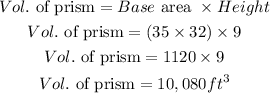
Find the volume of the topped pyramid

`\begin{gathered} Vol.\text{ of pyramid}=(BH)/(3) \\ Vol.\text{ of pyramid}=((35*32)*4)/(3) \\ Vol.\text{ of pyramid}=(4480)/(3) \\ Vol.\text{ of pyramid}=1493.3ft^3 \end{gathered}`

Determine the area of the tent

`\begin{gathered} volume\text{ of tent}=10,080+1493.3 \\ volume\text{ of tent}=11573.3ft^3 \end{gathered}`

Therefore the total volume of the tent to the nearest tenth is 11573.3 cubic feet