Question

The research department of a board game company surveyed some shoppers at a local mall. The team asked shoppers how often they read the newspaper, among other questions. Then the team played a memory card game with the shoppers. They recorded how many cards each shopper remembered. 1-3 cards 4-6 cards 0 times a week 8 9 1-2 times a week 15 10 What is the probability that a randomly selected shopper reads the newspaper 1-2 times a week or remembered 1-3 cards? Simplify any fractions.

Answer 1

Step 1: Write out the formula

`P(\text{A or B) = P(A) + P(B) - P(A}\cap B)`
`\begin{gathered} \text{where } \\ A\text{ and B are events} \end{gathered}`

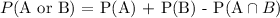
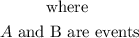
Step 2: Write out the given values and substitute them into the formula

Let A be the event of "shopper reads the newspaper 1-2 times.

and

Let B be the event of "shopper remembered 1-3 cards".

n(A) = 15 + 10 = 25

n(B) = 8 + 15 = 23

n(U) = 8 + 15 + 9 + 10= 42

`n(A\cap B)=15`

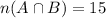
Therefore,

`\begin{gathered} P(A)=(25)/(42) \\ P(B)=(23)/(42) \\ P(A\cap B)=(15)/(42) \end{gathered}`

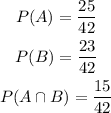
Hence,

`P(\text{A or B) = }(25)/(42)+(23)/(42)-(15)/(42)=(11)/(14)`

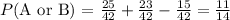
Thus the probability that a randomly selected shopper reads the newspaper 1-2 times a week or remembered 1-3 cards is 11/14