Question

Solve each system of equations algebraically.
`(y = {x}^(2) + 1 \\ (2x + y = 9`

Answer 1

For the system of equations:

`\begin{gathered} y=x^2+1 \\ 2x+y=9 \end{gathered}`

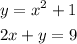
We can start by solving one of the equations for one of the variables and substituting into the other equation.

Since x has a quadratic term in the first equation, we can start by finding x first. To do this, we will need to substitute y and it is alredy solved in the first equation.

So, the first step is to substitute the first equation into the second:

`\begin{gathered} 2x+y=9 \\ 2x+x^2+1=9 \\ x^2+2x+1-9=0 \\ x^2+2x-8=0 \end{gathered}`

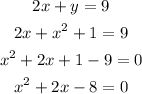
Now, we have a quadratic equation, so we can apply the quadratic formula to find its zeros:

`\begin{gathered} x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}=\frac{-2\pm\sqrt[]{(-2)^2-4\cdot1\cdot(-8)}}{2\cdot1} \\ x=\frac{-2\pm\sqrt[]{4+32}}{2}=\frac{-2\pm\sqrt[]{36}}{2}=(-2\pm6)/(2)=-(2)/(2)\pm(6)/(2)=-1\pm3 \\ x_1=-1+3=2 \\ x_2=-1-3=-4 \end{gathered}`

So, we have found two possible values for x.

Now, we need to find the corresponding values for y.

For x₁ = 2, we substitute it into either equations, let's do into the second:

`\begin{gathered} 2x+y=9 \\ 2\cdot2+y_1=9_{} \\ 4+y_1=9_{} \\ y_1=9-4 \\ y_1=5 \end{gathered}`

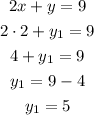
So, x₁ = 2 and y₁ = 5 is one of the solutions.

For x₂ = -4, we have:

`\begin{gathered} 2x+y=9 \\ 2\cdot(-4)+y_2=9 \\ -8+y_2=9 \\ y_2=9+8 \\ y_2=17 \end{gathered}`

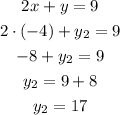
So, x₁ = -4 and y₁ = 17 is the second solution.

So, the solutions for this system of equations is (2, 5) and (-4, 17).