Question

After 3 minutes of exercise, Malcolm's heart rate is 80 beats per minute. After 5 minutes, itincreased to 94 beats per minute. Let a represent the number of minutes since Malcolm beganexercising, and let y represent Malcolm's heart rate (assume his heart rate increased at a constantrate). Write two linear equations (one in slope-intercept form and one in point-slope form) tomodel his heart rate. Then, calculate his heart rate after 12 minutes of exercise.♡14

Answer 1

1. i. The slope-intercept equation is:

`y=7x+59`

1. ii. The point-slope equation is:

`\begin{gathered} (y-80)=7(x-3) \\ OR \\ (y-94)=7(x-5) \end{gathered}`

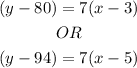
2. Malcolm's heart rate after 12 minutes is 143

SOLUTION

Problem Statement

The question tells us 3 minutes of exercise leads to 80 beats per minute with Malcolm's heart. The question goes on to say after 5 minutes, his beats per minute is 94 in magnitude.

We are asked to find two linear equations to model his heart rate.

Method

In order to solve this problem, we need to:

1. Calculate the slope (m) of the equation. To find the slope, we use the formula below:

`\begin{gathered} m=(y_2-y_1)/(a_2-a_1) \\ \text{where,} \\ m=\text{slope} \\ _{}y_2=\text{the second heart rate} \\ y_1=\text{the frist heart rate} \\ x_2=\text{second minutes passed} \\ x_1=\text{first minutes passed} \end{gathered}`

2. Find the value of the y-intercept of the linear equation.

3. Write out the heart-rate linear equation and find the value of the heart rate after 12 minutes

Implementation

1. Calculate the slope (m) of the equation. To find the slope, we use the formula below:

`\begin{gathered} y_2=94,x_2=5 \\ y_1=80,x_1=3 \\ \\ \therefore m=(94-80)/(5-3) \\ m=7 \end{gathered}`

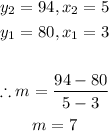
Thus, the slope is 7

2. Find the value of the y-intercept of the linear equation.

The y-intercept can be gotten by substituting the value of x and y into the general equation of a line.

The equation of a line is given by:

`\begin{gathered} y=mx+c \\ \text{where,} \\ m=\text{slope} \\ c=y-\text{intercept} \end{gathered}`

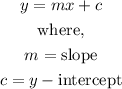
Once we have substituted, it becomes a matter of making the y-intercept the subject of the formula. The values of x and y we can use can either be: (3, 80) or (5, 94)

`\begin{gathered} \text{Given the general equation:} \\ y=mx+c \\ \text{choosing x=3 and y =80}.\text{ Remember that slope (m) = 7} \\ 80=3(7)+c \\ 80=21+c \\ \text{Subtract 21 from both sides} \\ 80-21=21-21+c \\ \therefore c=59 \end{gathered}`

Thus, the y-intercept is 59

3. Write out the heart-rate linear equation and find the value of the heart rate after 12 minutes

The slope-intercept form equation of Malcolm's heart rate is:

`\begin{gathered} y=mx+c \\ m=7,c=59 \\ \therefore y=7x+59 \end{gathered}`

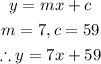
The point-slope form of Malcolm's heart rate is gotten using the formula given below:

`(y-y_1)=m(x-x_1)`

Thus, the point-slope equation is gotten using either (3, 80) or (5, 94):

`\begin{gathered} _{} \\ \text{ Using,} \\ x_1=3,y_1=80 \\ (y-80)=7(x-3) \\ \\ \text{using,} \\ x_2=5,y_2=94 \\ (y-94)=7(x-5) \\ \\ \therefore\text{The possible point-slope equations are:} \\ (y-80)=7(x-3) \\ (y-94)=7(x-5) \end{gathered}`

Using the slope-intercept form of the equation to calculate Malcolm's heart rate after 12 minutes. This means that the value of x to be used is 12 and we are trying to find y.

Thus, we have:

`\begin{gathered} y=7x+59 \\ \text{substituting x=12} \\ y=7(12)+59 \\ y=84+59 \\ \therefore y=143 \end{gathered}`

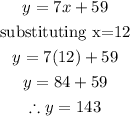
Thus, his heart rate after 12 minutes is 143

Final Answer:

To recap,

1. i. The slope-intercept equation is:

`y=7x+59`

1. ii. The point-slope equation is:

`\begin{gathered} (y-80)=7(x-3) \\ OR \\ (y-94)=7(x-5) \end{gathered}`

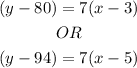
2. Malcolm's heart rate after 12 minutes is 143