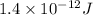Answer: The binding energy per nucleon is
Step-by-step explanation:
Nucleons are defined as the sub-atomic particles which are present in the nucleus of an atom. Nucleons are protons and neutrons.
We are given a nucleus having representation:
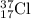
Number of protons = 17
Number of neutrons = 37 - 17 = 20
To calculate the mass defect of the nucleus, we use the equation:
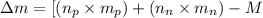
where,
 = number of protons = 17
= number of protons = 17
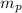 = mass of one proton = 1.00728 amu
= mass of one proton = 1.00728 amu
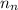 = number of neutrons = 20
= number of neutrons = 20
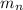 = mass of one neutron = 1.00866 amu
= mass of one neutron = 1.00866 amu
M = nuclear mass = 36.9566 amu
Putting values in above equation, we get:
![\Delta m=[(17* 1.00728)+(20* 1.00866)]-36.9566\\\\\Delta m=0.34036amu](https://img.qammunity.org/2018/formulas/chemistry/high-school/kl27vri85sgf6vi1ig28cbdh7pvcy1k8wl.png)
To calculate the binding energy of the nucleus, we use the equation:

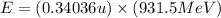 (Conversion factor:
(Conversion factor:
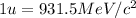 )
)
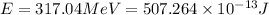 (Conversion factor:
(Conversion factor:
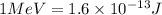 )
)
Number of nucleons in
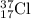 atom = 37
atom = 37
To calculate the binding energy per nucleon, we divide the binding energy by the number of nucleons, we get:
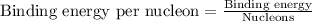
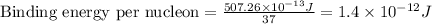
Hence, the binding energy per nucleon is