Question

Consider the graph of the polynomial function p(x)=(x-1)(x+2)(x+5)

Answer 1

what's the question?

(if its x-intercepts, its 1,-2,-5 since you use the zero product property)

Answer 2

The graph is first increasing then decreasing to right.

Explanation:

Given : The polynomial function
`p(x)=(x-1)(x+2)(x+5)`

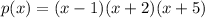
To find : Consider the graph of the polynomial function and the graph is ?

Solution :

First we graph the given polynomial function,

`p(x)=(x-1)(x+2)(x+5)`

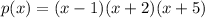
which cut the x-axis at 1,-2,-5.

Refer the attached figure below.

The graph is first increasing to the point (-3.7,10.3) then decreasing to point (-0.268,-10.392) then increasing to infinity.

Therefore, The graph is first increasing then decreasing to right.