Question

What is the area of the sector with the 90- degree angle?What is the area of the blue region?

Answer 1

To answer this question, we need:

1. Find the area of the sector with a 90-degree angle.

2. Find the area of the triangle in the figure.

3. Subtract the area of the 90-degree angles minus the area of the triangle.

We can proceed as follows:

Area of the sector with a 90-degree angle

To find it, we can use the next formula:

`A_{\text{sector}}=(n)/(360)\cdot\pi\cdot r^2`

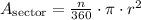
Where, in this case, n = 90 (number of degrees in central angle of the sector). We also have that r = 16 units (the radius of the circle). Now, we can calculate this area:

`A_{\text{sector}}=(90)/(360)\cdot\pi\cdot(16)^2=(1)/(4)\cdot\pi\cdot256=64\pi\approx201.0619\ldots`

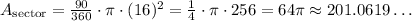
The area, in this case, is 64pi square units or 201.0619 square units.

Area of the triangle in the figure

Since this is a right triangle, we have that the base = 16 units, and the height is equal to 16 too. Then, we have:

`A_{\text{triangle}}=(b\cdot h)/(2)=(16\cdot16)/(2)=(256)/(2)\Rightarrow A_(triangle)=128`

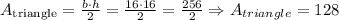
Now, we have that the area of the triangle is 128 square units.

Area of the Blue Region

This area is:

`A_{\text{sector}}-A_{\text{triangle}}=201.0619-128=73.0619`

Therefore, the area of the blue region is, approximately, equals to 73.0619 square units.