Question

What are the wavelength limits of the audible range of the sound spectrum? (Use the speed of sound in air. The speed of sound in air is 344 m/s. The audible range of the sound spectrum contains frequencies as low as 30 Hz and as high as 25 kHz.) smallest value answer in:____m largest value answer in:____m

Answer 1

Given:

Speed of sound, v = 344 m/s

Low frequency, fl = 30 Hz.

High frequency, fh = 25 kHz.

Let's find the audible range of sound spectrum.

The range of sound can be said to be the wavelength.

To find the wavelength, apply the formula:

`\lambda=(v)/(f)`

Where:

• λ is the wavelength in meters (m).

,

• v is the speed in meters per second (m/s)

,

• f is the frequency (Hz.)

• To find the largest wavelength, we have:

`\begin{gathered} \lambda_L=(v)/(f_s) \\ \\ \lambda_L=(344)/(30) \\ \\ \lambda_L=11.47\text{ m} \end{gathered}`

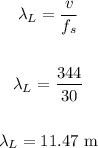
• To find the smallest wavelength, we have:

`\begin{gathered} \lambda_s=(v)/(f_h) \\ \\ \lambda_s=(344)/(25*10^3) \\ \\ \lambda_s=0.014\text{ m} \end{gathered}`

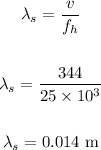
Therefore, we have:

Smallest value: 0.014 m

Largest value: 11.47 m

ANSWER:

• Smallest value: , 0.014 m

,

• Largest value: , 11.47 m