Answer: The other point is (17, -6).
Explanation:
Midpoint (x,y) of the line segment joining (a,b) and (c,d) is given by :-
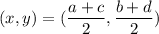
Here, we need to find the other endpoint of a segment with one endpoint at(-3, 8) and the midpoint at (7, 1).
Let other point be (a,b), then
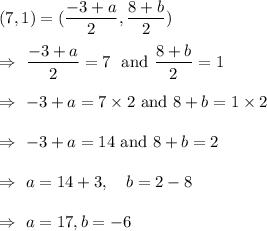
hence, the other point is (17, -6).