Question

Is this sequence geometric? If so, identify the common ratio.

1/4, 3/16, 9/64, 27/256, 81/1024, ...

a) yes; 1/3
b) yes; 3/4
c) not geometric
d) yes; 2/9

Answer 1

Yes, it is geometric and the ratio is 3/4. You can tell because you can divide each one by the one before and you get 3/4. (3/16)/(1/4)=3/4, (9/64)/(3/16)=3/4, and so on.

Hope that helps

Answer 2

Answer: The correct option is (b) Yes,
`(3)/(4).`

Step-by-step explanation: The given sequence is

`(1)/(4),~(3)/(16),~(9)/(64),~(27)/(256),~(81)/(1024),~.~.~.`

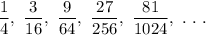
We are to check whether the given sequence is geometric. If so, we are to find the common ration.

GEOMETRIC SEQUENCE: A sequence of numbers where each term is found by multiplying by a constant with the preceding term. This constant is called the common ratio, r.

In the given sequence, we can see

`((3)/(16))/((1)/(4))=(3)/(4)~~~~\Rightarrow (3)/(16)=(3)/(4)* (1)/(4),\\\\\\((9)/(64))/((3)/(16))=(3)/(4)~~~~\Rightarrow (9)/(64)=(3)/(4)* (3)/(16),\\\\\\((27)/(256))/((9)/(64))=(3)/(4)~~~~\Rightarrow (27)/(256)=(3)/(4)* (9)/(64),\\\\\\((81)/(1024))/((27)/(256))=(3)/(4)~~~~\Rightarrow (81)/(1024)=(3)/(4)* (27)/(256),~etc`

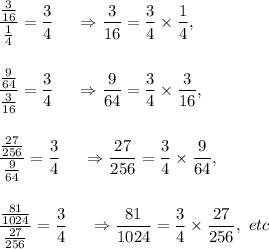
Therefore, each term is found by multiplying
`(3)/(4)` with the preceding term.

Thus, the given sequence is geometric and its common ratio is
`(3)/(4)`

Option (b) is correct.