Question

Ninety six passengers rode a train. Tickets for regular coach seats are $114. Tickets for sleeper car seats are $295. The receipts for the trip totaled $19,994. How many passengers purchased each type of ticket ?

Answer 1

c = amount of folks buying coach tickets
s = amount fo folks buying sleeper car tickets

we know the train had a total of 96 passengers, so, whatever "c" and "s" are
c + s = 96

we know all tickets sold for all 96 passengers was 19994, so, if "c" folks rode coach, each ticket is 114, thus 114*c is how much money was from couch, or 114c

if "s" folks rode the sleeper car, we know each ticket is 295 each, so 295*s is how much money was from the sleeper car

we also know, the total for all tickets is 19994

thus 114c + 295s = 19994

thus
`\bf \begin{cases} c+s=96\implies \boxed{s}=96-c\\ 114c+295s = 19994\\ ----------\\ 114c+295\left( \boxed{96-c} \right)=19994 \end{cases}`

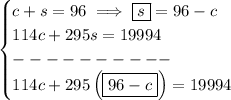
solve for "s", to see how many folks rode coach

what about the sleeper car? well, s = 96 - c