Question

The coordinates of the vertices of △JKL are J(0, 2) , K(3, 1) , and L(1, −5) .

Drag and drop the choices into each box to correctly complete the sentences.

Answer 1

we know that

If two lines are perpendicular, then the product of their slopes is equal to minus one

so

`m1*m2=-1`

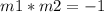
we have

`J(0,2)\\K(3,1)\\L(1,-5)`

Remember that

the formula to calculate the slope between two points is equal to

`m=((y2-y1))/((x2-x1))`

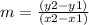
Step 1

Find the slope JK

we have

`J(0,2)\\K(3,1)`

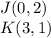
substitute in the slope's formula

`m=((1-2))/((3-0))`

`m=((-1))/((3))`

`mJK=-(1)/(3)`

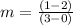
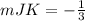
Step 2

Find the slope KL

we have

`K(3,1)\\L(1,-5)`

substitute in the slope's formula

`m=((-5-1))/((1-3))`

`m=((-6))/((-2))`

`mKL=3`

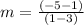
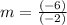
Step 3

Find the slope JL

we have

`J(0,2)\\L(1,-5)`

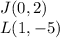
substitute in the slope's formula

`m=((-5-2))/((1-0))`

`m=((-7))/((1))`

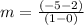
`mJL=-7/tex]</p><p><strong>Step 4</strong></p><p><u>Verify if the sides of the triangle are perpendicular</u></p><p>Multiply the slopes</p><p><strong>JK and KL</strong></p><p>[tex]mJK=-(1)/(3)`

`mKL=3`

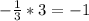`-(1)/(3)*3=-1` ------> sides JK and KL are perpendicular

when verifying that it has two perpendicular sides, it is not necessary to continue verifying the others, since a triangle can only have a single right angle

therefore

the answer in the attached figure