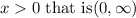Question

The length of a rectangle is 4 units greater than its width, and the area of the rectangle can be expressed by the equation y = x2 + 4x. What is a reasonable domain for this function?

Answer 1

hello :
a reasonable domain for this function is : ]0 ; + ∞[
because : x > 0 and x+4 > 0 .... f(x) = x(x+4) ... length ×width

Answer 2

All values of x greater than 0.

Explanation:

Let x represent width of rectangle.

We have been given that the length of rectangle is 4 units greater than its width. So the length of rectangle would be
`x+4`.

The domain of a function is all real values of independent variable that the function can take on.

Since our given function represents the area of rectangle, where x represents width of rectangle, so the value of x should be a non zero and non-negative number.

Therefore, the domain of our given function would be
`x>0\text{ that is}(0,\infty)`