Question

For which function is f(x) not equal to f-1(x)?

f(x)= 2-x
f(x)=2/x
f(x)=-x
f(x)=2x

Answer 1

Answer: The correct option is (D)
`f(x)=2x.`

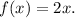
Step-by-step explanation: We are given to select the correct function for which f(x) is not equal to
`f^(-1)(x).`

Option (A) :

Here,

`f(x)=2-x.`

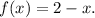
Let f(x) = y, so
`x=f^(-1)(y).`

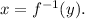
Therefore,

`f(x)=2-x\\\\\Rightarrow y=2-f^(-1)(y)\\\\\Rightarrow f^(-1)(y)=2-y\\\\\Rightarrow f^(-1)(x)=2-x.`

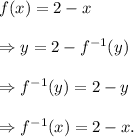
So, the function is equal to its inverse.

This option is incorrect.

Option (B) :

Here,

`f(x)=(2)/(x).`

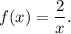
Therefore,

`f(x)=(2)/(x)\\\\\Rightarrow y=(2)/(f^(-1)(y))\\\\\Rightarrow f^(-1)(y)=(2)/(y)\\\\\Rightarrow f^(-1)(x)=(2)/(x).`

So, the function is equal to its inverse.

This option is incorrect.

Option (C) :

Here,

`f(x)=-x.`

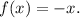
Therefore,

`f(x)=-x\\\\\Rightarrow y=-f^(-1)(y)\\\\\Rightarrow f^(-1)(y)=-y\\\\\Rightarrow f^(-1)(x)=-x.`

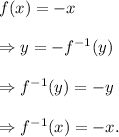
So, the function is equal to its inverse.

This option is incorrect.

Option (D) :

Here,

`f(x)=2x.`

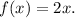
Therefore,

`f(x)=2x\\\\\Rightarrow y=2f^(-1)(y)\\\\\Rightarrow f^(-1)(y)=(y)/(2)\\\\\Rightarrow f^(-1)(x)=(x)/(2).`

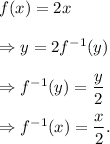
So, the function is NOT equal to its inverse.

This option is correct.

Thus, (D) is the correct option.

Answer 2

In the given function f(x) is not equal to f-1(x), if the given problem is asking for the not equal to inverse, then we have the solution below:
f(x)=2x
f-(x)=x/2
The answer is letter "B" which is f(x)=2/x.