Question

The density of no2 in a 3.50 l tank at 780.0 torr and 37.0 °c is __________ g/l.

Answer 1

The density of nitrogen dioxide in a 3.50 L tank at 780.0 Torr and 37.0 °C is 1.86 g/L.

Step-by-step explanation:

To calculate the density of gas, we use the equation given by ideal gas equation:

`PV=nRT`

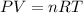
Number of moles (n)

can be written as:
`n=(m)/(M)`

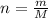
where, m = given mass

M = molar mass

`PV=(m)/(M)RT\\\\PM=(m)/(V)RT`

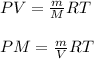
where,

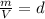`(m)/(V)=d` which is known as density of the gas

The relation becomes:

`PM=dRT` .....(1)

We are given:

M = molar mass of argon = 46 g/mol

R = Gas constant =
`0.0821\text{ L atm }mol^(-1)K^(-1)`

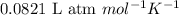
T = temperature of the gas =
`27.0^oC=[37.0+273]K=310 K`

P = pressure of the gas = 780.0 Torr=
`(780.0 )/(760) atm=1.03 atm`

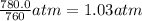
1 atm = 760 Torr

Putting values in equation 1, we get:

`1.03 atm* 46 g/mol=d* 0.0821\text{ L atm }mol^(-1)K^(-1)* 310  K\\\\d=1.86 g/L`

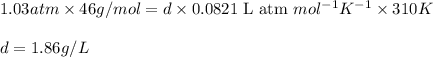
The density of nitrogen dioxide in a 3.50 L tank at 780.0 Torr and 37.0 °c is 1.86 g/L.

Answer 2

We assume that the given gas, NO2, is an ideal gas such that we will be able to use the equation,
n/V = P/RT
where n is the number of moles, V is volume, P is pressure, R is gas constant, and T is temperature in kelvin. Substituting the known values,
n/V = (1 atm) / (37 + 273)(0.0821 L.atm/mol.K)
n/V = 0.039 mol/L
Converting this to density by multiplying the value with the molar mass,
density = (0.039 mol/L) x (46 g/mol) = 1.8 g/L