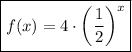Question

Which exponential function is represented by the values in the table?

Answer 1

`\displaystyle f(x)=4\cdot\left((1)/(2)\right)^x`

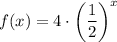
Explanation:

Exponential Function

The exponential function can be written with the general equation:

`f(x)=A\cdot r^x`

Where A is the value when x=0 and r>0 is the ratio. If r is greater than 1, the function is increasing, if r is less than 1, the function is decreasing.

The table shows the relation between values of x and values of the function y. Note that as x increases (one by one), y decreases with a ratio of 1/2. Only the last two choices have ratios of r=1/2. We only have to test which one of them has the correct value of y=4 when x=0.

Substituting in the third function:

`\displaystyle f(x)=4\cdot\left((1)/(2)\right)^x`

`\displaystyle f(0)=4\cdot\left((1)/(2)\right)^0`

`f(0) = 4`

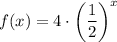
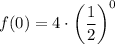
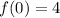
This gives the correct value of f(0)=4.

Substituting in the fourth function:

`\displaystyle f(x)=(1)/(2)\cdot\left((1)/(2)\right)^x`

`\displaystyle f(0)=(1)/(2)\cdot\left((1)/(2)\right)^0`

`f(0) = (1)/(2)`

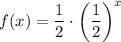
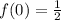
This choice is wrong

Correct choice:

`\boxed{\displaystyle f(x)=4\cdot\left((1)/(2)\right)^x}`