Answer:
width of the garden's is, g-6 unit
Explanation:
Area of rectangle(A) is given by:
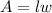
where
l is the length of the rectangle
w is the width of the rectangle.
As per the statement:
The area of a garden is given by the trinomial of
 and the garden's length is g+4
and the garden's length is g+4
⇒
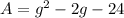 square units and
square units and
 units
units
Factorize

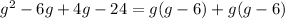
⇒
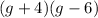
⇒A =
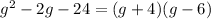
Substitute these in [1] we have;
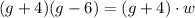
Divide both sides by g+4 we have;
⇒

Therefore, the width of the garden's is, g-6 unit