Question

3. If sin(θ ) = −1/4 and θ is in the third quadrant, find cos(θ ).

Answer 1

cosθ = -√15/4

STEP - BY - STEP EXPLANATION

What to find?

cos(θ )

Given:

sin(θ ) = −1/4

To solve the given problem, we will follow the steps below:

Step 1

Recall the trigonometric identities.

cos²θ + sin²θ = 1

Step 2

Substitute the value of sinθ into the above.

cos²θ + (-1/4)² = 1

cos²θ + 1/16 = 1

Step 3

Subtract 1/16 from both-side of the equation.

cos²θ = 1 - 1/16

Step 4

Simplify the right-hand side of the equation.

`\cos ^2\theta=(16-1)/(16)`
`\cos ^2\theta=(15)/(16)`

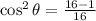
Step 5

Take the square root of both-side of the equation.

`\cos \theta=\pm\sqrt[]{(15)/(16)}`
`\cos \theta=\pm\frac{\sqrt[]{15}}{4}`

Since θ is in the third quadrant;

We can see that in the third quadrant, only tanθ is positive, cosθ is negative.

Hence, we will pick only the negative value.

Therefore,

`\cos \theta=\frac{-\text{ }\sqrt[]{15}}{4}`