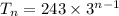Question

A geometric sequence is defined by a the recursive formula t1 = 243, tn + 1 = tn 3 , where n ∈N and n ≥ 1. The general term of the sequence is

Answer 1

`t_(n)=243*3^((n-1))`×

Answer 2

`T_n = 243 * 3^(n-1)`

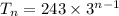
Explanation:

Given :
`t_1=243`

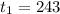
Expression :
`t_(n+1)=3t_n`

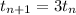
Put n = 1

`t_(1+1)=3* t_1`

`t_(2)=3* 243`

`t_(2)=729`

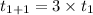
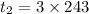
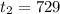
Put n = 2

`t_(2+1)=3* t_2`

`t_(3)=3*729`

`t_(3)=2187`

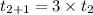
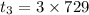
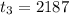
Put n = 3

`t_(3+1)=3* t_3`

`t_(4)=3*2187`

`t_(4)=6561`

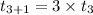
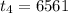
Continuing in this manner we will get the geometric sequence :

243,729,2187,6561 ........

Formula for nth term of G.P. :
`T_n = ar^(n-1)`

a= 243

r = common ratio between the consecutive terms .

`r=(729)/(243)=(2187)/(729)=3`

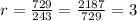
Substituting values in the formula :

`T_n = 243 * 3^(n-1)`

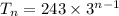
Hence the general term of the sequence is
`T_n = 243 * 3^(n-1)`