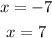A)
The equation given, is
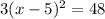
To solve for x, we first divide both sides by '3',
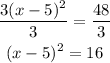
Now, we take the square root and solve for the value(s) of x:
![\begin{gathered} \sqrt[]{(x-5)^2}=\pm\sqrt[]{16} \\ x-5=\pm4 \\ x=\pm4+5 \\ x=1,9 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/iwfp2zvqtrrklrkjmr4d.png)
Thus, the solutions are

B)
The equation to solve:

Let's solve for x. The algebra is shown below:
![\begin{gathered} 2x^2-56=42 \\ 2x^2=42+56 \\ 2x^2=98 \\ x^2=49 \\ \sqrt[]{x^2}=\pm\sqrt[]{49} \\ x=7,-7 \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/q5g308ggwrnx2ii4y71o.png)
Thus, the solutions are