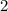we know that
A relationship between two variables, x, and y, represent an inverse variation if it can be expressed in the form

in this problem we have that
the value of the constant K is equal to
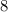
so
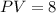
Solve for V
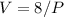
Find the value of V for
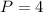
substitute the value of P in the formula
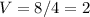
therefore
the answer is
the value of V is equal to