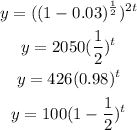All the function as exponential and they can be written in the form:
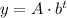
When written in thif form, we can check if the function describe an exponential growth or decay by checking "b".
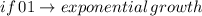
Let's start with the two that are already in this form:
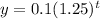
Here, b = 1.25. Since b > 1, this describe an exponential growth.
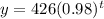
b = 0.98. Since 0 < b < 1, this describe an exponential decay.
Now, this one is almost in this form:
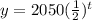
We just need to turn b = 1/2 to decimal to be sure. 1/2 is 0.5, so b = 0.5. Since 0 < b < 1, this describe an exponential decay.
This one, we need to evaluate the expression inside parenthesis:
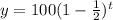
Here, b is:

Since 0 < b < 1, this describe an exponential decay.
Lastly,, we have the following:
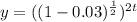
First, le'ts make the substraction:

Now, notice that when we have a multiplication in an exponent, we can do the following:
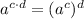
Thus, we can do the contrary. So,
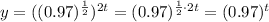
Notice that we don't have anything in the place of "A", but this means that A = 1. This doesn't change b, that is, in this case equal to 0.97. Since 0 < b < 1, this describe an exponential decay.
So, the function that describe exponential growth is:
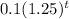
And the functions that describe exponential decay are: