Question

Part of a payment of $40,000 was invested at 10% annual interest, and the rest was invested at 8% annual interest. If the total annual interest is $3500, how much was invested at the 8% rate?

Answer 1

Answer: 25,000

Step-by-step explanation:

Let's call X the part of the payment invested at 10% annual and Y the part of the payment invested at 8% annual interest.

Since the total payment is 40,000, we can write the following equation:

X + Y = 40,000

On the other hand, the annual interest can be calculated as:

I = P*r

Where P is the initial value and r is the annual interest rate

Then, the total annual interest can be calculated as:

I = X*0.1 + Y*0.08

Since the total annual interest is $3500, we can write the following equation:

3500 = 0.1X + 0.08Y

Now, we can solve for X in the first equation and replace it on the second as:

`\begin{gathered} X=40,000-Y \\ 3500=0.1(40,000-Y)+0.08Y \end{gathered}`

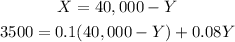
Solving for Y, we get:

`\begin{gathered} 3500=0.1\cdot40,000-0.1\cdot Y+0.08Y \\ 3500=4000-0.1Y+0.08Y \\ 3500=4000-0.02Y \\ 3500-4000=-0.02Y \\ -500=-0.02Y \\ (-500)/(-0.02)=Y \\ 25,000=Y \end{gathered}`

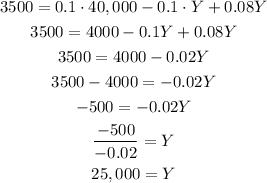
Therefore, 25,000 was invested at the 8% rate