Question

)) Which of these equations has no solutions? -3(x - 1) = 2x + 4 - 5x 2(10x – 3) = 2 + 5x - 7/ 2(2x - 1) = x + 4 )) Which statement explains a way you can tell the equation has no solutions? It is equivalent to an equation that has the same variable terms but different constant terms on either side of the equal sign. It is equivalent to an equation that has the same variable terms and the same constant terms on either side of the equal sign. It is equivalent to an equation that has different variable terms on either side of the equation.

Answer 1

Given the equations:

`\begin{gathered} -3(x-1)=2x+4-5x \\ \\ (1)/(2)(10x-3)=2+5x-(7)/(2) \\ \\ 2(2x-1)=x+4 \end{gathered}`

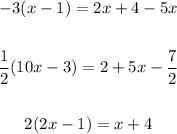
Let's evaluate each equation.

`\begin{gathered} -3(x-1)=2x+4-5x \\ \\ -3x+1=2x+4-5x \\ \\ -3x-2x+5x=4-1 \\ \\ 0=3 \\ \\ \text{This equation has no solution} \end{gathered}`
`\begin{gathered} (1)/(2)(10x-3)=2+5x-(7)/(2) \\ \\ 5x-(3)/(2)=2+5x-(7)/(2) \\ \\ \text{Multiply through by 2 to eliminate the fraction:} \\ 5x(2)-(3)/(2)\ast2=2(2)+5x(2)-(7)/(2)\ast2 \\ \\ 10x-3=4+10x-7 \\ \\ 10x-10x=4-7+3 \\ \\ 0=0 \\ \\ This\text{ equation has infinitely many solutions} \end{gathered}`
`\begin{gathered} 2(2x-1)=x+4 \\ \\ 4x-2=x+4 \\ \\ \text{Subtract x from both sides:} \\ 4x-x-2=x-x+4 \\ \\ 3x-2=4 \\ \\ \text{Add 2 to both sides:} \\ 3x-2+2=4+2 \\ \\ 3x=6 \\ \\ \text{Divide both sides by 3:} \\ (3x)/(3)=(6)/(3) \\ \\ x=2 \\ \\ \text{This equation has one solution} \end{gathered}`

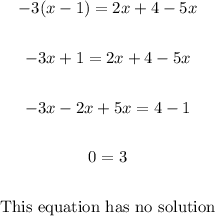
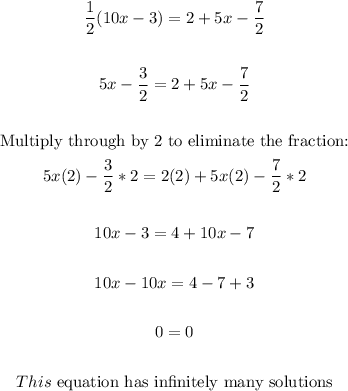
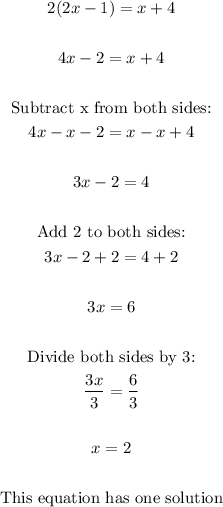
The statement that explains a way you can tell the equation has no solutions is:

It is equivalent to an equation that has the same variable terms but different constant terms on either side of the equal sign.

ANSWER:

Equation that has no solution: -3(x-1)=2x+4-5x

It is equivalent to an equation that has the same variable terms but different constant terms on either side of the equal sign.