Question

Instructions: Given the quadratic function, state whether the parabola opens up or down, and whether it has a maximum or minimum.

Answer 1

Answer:

The parabola opens down and has a vertex of (1, 7) which is a maximum value

Step-by-step explanation:

Given:

`y\text{ = -3x}^2\text{ + 6x + 4}`

To find:

a) if the parabola opens up or down

b) the coordinate of the vertex

c) If it is maximum or minimum

a) To determine if the parabola opens up or down, we will use the sign of the leading coefficient.

The leading coefficient 3x² is negative. when the sign is negative, it will open down. if positive, it will open up. Hence, the parabola opens down

b) To get the vertex of the parabola, we will apply the formula:

`\begin{gathered} Vertex\text{ = \lparen h, k\rparen} \\ h\text{ = }(-b)/(2a) \\ k\text{ = f\lparen}-(b)/(2a))\text{ = f\lparen h\rparen} \end{gathered}`
`\begin{gathered} from\text{ the quadratic equation: -3x}^2\text{ + 6x + 4} \\ a\text{ = -3, b = 6, c = 4} \\ h\text{ = }(-6)/(2(-3)) \\ h\text{ = }(-6)/(-6)\text{ = 1} \\ \\ k\text{ = f\lparen1\rparen} \\ we\text{ }will\text{ substitute for x in the given function } \\ f(1)\text{ = -3\lparen1\rparen}^2\text{ + 6\lparen1\rparen + 4} \\ f(1)\text{ = -3 + 6 + 4 = 7} \\ k\text{ = 7} \\ \\ Hence,\text{ the vertex \lparen h, k\rparen = \lparen1, 7\rparen} \end{gathered}`

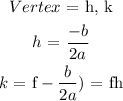
c) If a parabola opens up, the vertex will be the lowest value (minimum). If a parabola opens down, the vertex will be the highest value (maximum)>

Since our graph opens down, it has a maximum value

The parabola opens down and has a vertex of (1, 7) which is a maximum value