Answer:

Explanation:
The area of a triangle is found using the formula:
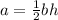
We know the area is 242 square feet and the base is 44 feet.
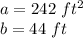
Substitute the values into the formula.
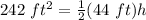
Multiply 1/2 and 44 or divide by 2.
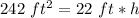
Since we are solving for h, we must isolate the variable. It is being multiplied by 22 and the inverse operation of multiplication is division. Divide both sides of the equation by 22.
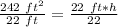
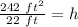
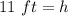
The height of the triangle is 11 feet