Question

The measure of two complementary angles are in ratio 2:3. What is the measure of the smaller angle

Answer 1

36°

Step-by-step explanation

Let a and b be the measures of the two angles. We know that they are complementary, so their measures add up to 90°. Also, we know that the quotient between their measures is 2/3,

`\begin{gathered} a+b=90 \\ (a)/(b)=(2)/(3) \end{gathered}`

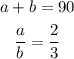
Solve the second equation for a,

`a=(2)/(3)b`

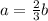
Replace a with this expression in the first equation,

`(2)/(3)b+b=90`

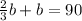
Add like terms,

`(5)/(3)b=90`

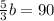
Solving for b,

`b=90\cdot(3)/(5)=54`

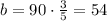
So the other angle is,

`a=(2)/(3)b=(2)/(3)\cdot54=36`

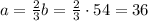
Hence, the measure of the smaller angle is 36°.