Answer:
The axis of symmetry is at
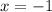
The graph has an x-intercept at
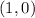
The graph has a vertex at
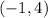
Explanation:
we have

Statements
case 1) The graph has root at
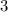 and
and

The statement is False
Because, the roots of the quadratic equation are the values of x when the value of y is equal to zero (x-intercepts)
Observing the graph, the roots are at
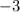 and
and

case 2) The axis of symmetry is at
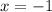
The statement is True
Observing the graph, the vertex is the point
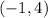
The axis of symmetry in a vertical parabola is equal to the x-coordinate of the vertex
so
the equation of the axis of symmetry is
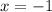
case 3) The graph has an x-intercept at
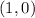
The statement is True
see procedure case 1)
case 4) The graph has an y-intercept at
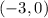
The statement is False
Because, the y-intercept is the value of y when the value of x is equal to zero
Observing the graph, the y-intercept is the point

case 5) The graph has a relative minimum at
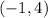
The statement is False
Because, is a vertical parabola open downward, therefore the vertex is a maximum
The point
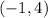 represent the vertex of the parabola, so is a maximum
represent the vertex of the parabola, so is a maximum
case 6) The graph has a vertex at
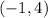
The statement is True
see the procedure case 5)
see the attached figure to better understand the problem