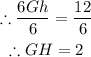Question

The quadrilaterals are similar if CB = 6 , 3, and CD = 4. find GH

Answer 1

Since ABCD and EFGH are similar

∴ Their corresponding sides are proportion (have equal ratios)

`\therefore(AB)/(EF)=(BC)/(FG)=(CD)/(GH)=(AD)/(HE)`

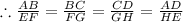
∵ BC = 6 and FG = 3

∵ CD = 4

→ Substitute then in the 2nd and 3rd ratio above to find GH

`\because(6)/(3)=(4)/(GH)`

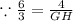
→ By using cross multiplication

∴ 6 x GH = 3 x 4

∴ 6GH = 12

→ Divide both sides by 6 to find GH

`\begin{gathered} \therefore(6Gh)/(6)=(12)/(6) \\ \therefore GH=2 \end{gathered}`