Answer:
Option (B) is correct i.e the amount of interest accrued on the second account is double the amount of interest accrued on the first account.
Explanation:
As given
The amount of simple interest accrued on a sum of money varies jointly with the amount of money, the interest rate, and the time the money is invested.
Thus
Formula for simple interest .
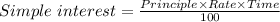
As given
A sum of money is invested at 4% for 3 years and accrues $168 in interest.
Put in the above
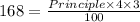
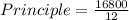
Principle = $1400
As given
The same sum of money is invested in a second account at 6% for 4 years.
Put in the formula

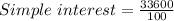
Simple interest = $336
Thus Option (B) is correct i.e the amount of interest accrued on the second account is double the amount of interest accrued on the first account.